Cumulative Distribution Function

Cumulative Distribution Function
Description	The Cumulative Distribution Function (CDF) helps to learn about the distribution of data by calculating the cumulative probability of a given variable value in a dataset.
Why to use	To calculate the probability of a given variable value by trying to fit it with different distribution functions.
When to use	For numerical variables having positive or negative values.	When not to use	—
Prerequisites	The data should not contain any missing values.
Input	Numerical variable having any positive or negative value.	Output	CDF curve Cumulative Probability Function predicted by CDF
Statistical Methods used	Cumulative Probability	Limitations	It can be used only on numerical data.

Cumulative Distribution Function is located under Model Studio () in Statistical Analysis, in the left task pane. Use the drag-and-drop method to use the algorithm in the canvas. Click the algorithm to view and select different properties for analysis.

Refer to Properties of Cumulative Distribution Function.

CDF takes input as a random variable value (either discrete or continuous) and then determines the cumulative probability of that variable. By default, the data is sorted and then sent to the algorithm. Also, in the output Data tab, the resultant data appears in a sorted manner.

Properties of Cumulative Distribution Function

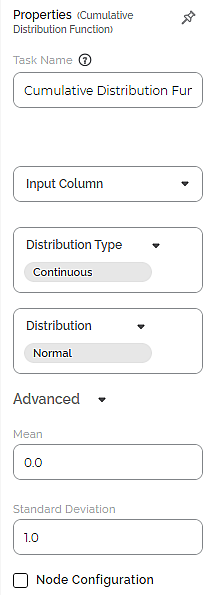
The available properties of the Cumulative Distribution Function are as shown in the figure below.

The table below describes the different fields present on the Properties pane of the Cumulative Distribution Function.

Table: Description of Fields present on the Properties Pane of Cumulative Distribution Function

Field		Description	Remark
Task Name		It is the name of the task selected on the workbook canvas.	You can click the text field to edit or modify the name of the task as required.
Input Column		It allows you to select the variable to be selected as the input attribute.	You can select any numerical type of variable. If the data contains missing values, you have to impute the missing values before using the Cumulative Distribution Function.
Distribution Type		It allows you to select the type of distribution to be applied to the data.	There are two types of Distribution to select from: Continuous Discrete
Distribution		It allows you to select the sub-type of the Distribution selected above.	The sub-types present in Continuous and Discrete Distribution are given in Description of Advanced Options for Distribution Type and Distribution Pairs.
Advanced	Node Configuration	It allows you to select the instance of the AWS server to provide control on the execution of a task in a workbook or workflow.	For more details, refer to Worker Node Configuration.

In the Advanced options, algorithmic parameters for CDF also appear according to the pair of Distribution Type and Distribution selected. These parameters change according to the Distribution Type and Distribution selected.

For example, when you select the Cumulative Distribution Function node, the option for Distribution Type is Continuous and that for Distribution is Normal. In this case, the parameters that appear in the Advanced Options are Mean and Standard Deviation.

The table below describes these two parameters.

Table: Description of Advanced Options for Continuous Distribution Type and Normal Distribution

Field	Description	Remark
Mean	It allows you to select the mean value corresponding to the normal distribution.	It appears by default when the Distribution Type is Continuous, and Distribution is Normal. The default value for Mean is 0.0.
Standard Deviation	It allows you to select the value of standard distribution corresponding to the normal distribution.	It appears by default when the Distribution Type is Continuous, and Distribution is Normal. The default value for Standard Deviation is 1.0.

The table below gives the other parameters that appear in advanced options available for individual Distribution Types and Distribution pairs.

Distribution Type	Distribution	Parameter in Advanced Options	Description
Continuous	Chi-squared	Degrees of Freedom	The default value is 1. You can select the minimum and maximum values (integers) as 1 and 30, respectively.
	Standard Exponential	—	The applicable parameters are already configured.
	Exponential	Alpha (α)	You can select any real float value greater than zero.
	F-distribution	Degree of Freedom 1	The default value for both the Degrees of Freedom is 1.
	F-distribution	Degree of Freedom 2	The default value for both the Degrees of Freedom is 1.
	Gamma	Alpha (α)	The default value is 1. You can select any integer value greater than zero.
	Standard Normal	—	The applicable parameters are already configured.
	Normal	Mean	The default value is 0.0. The value for mean lies in the range from − ∞ to ∞ (minus infinity to infinity).
	Normal	Standard Deviation	The default value is 1.0. You can select any real number strictly greater than zero.
	t	Degrees of Freedom	The default value is 1. You can select the minimum and maximum values (integers) as 1 and 30, respectively.
	Standard Uniform	—	The applicable parameters are already configured.
	Continuous Uniform	Lower Limit	The default value is 1.
	Continuous Uniform	Upper Limit	The default value is 2. Upper limit should always be greater than lower limit value. Both the upper and lower limit values lie in the range from − ∞ to ∞ (minus infinity to infinity).
	Weibull_min	Shape Parameter	The default value is 1. You can select any value greater than zero.
	Weibull_max	Shape Parameter	The default value is 1. You can select any value greater than zero.
Discrete	Bernoulli	Probability	The default value is 0.5. You can select the minimum and maximum values (float) as 0 and 1, respectively.
	Binomial	Number of trials	The default value is 10. You can select the minimum value as zero (0). You can select any real value greater than zero (as any value above 0 can be selected as maximum value)
	Binomial	Probability	The default value is 0.5. You can select the minimum and maximum values (float) as 0 and 1, respectively.
	Geometric	Probability	The default value is 0.5. You can select the minimum and maximum values (float) as 0 and 1, respectively.
	Hypergeometric	Population Size	The default value is 10. You can select the minimum value as zero (0). Any natural number can be selected as population size.
		Number of successes	The default value is 5. You can select the minimum value as zero (0). You can select any value up to the population size as the maximum value.
		Number of draws	The default value is 5. You can select the minimum value as zero (0). You can select any value up to the population size as the maximum value.
	Poisson	lambda	The default value is 5. You can select any value greater than zero as the minimum value. You can select any float value (above the minimum value) as the maximum value (as any value above 0 can be selected as maximum value)
	Discrete Uniform	Lower Limit	The default value is 1.
	Discrete Uniform	Upper Limit	The default value is 2.

Example of Cumulative Distribution Function

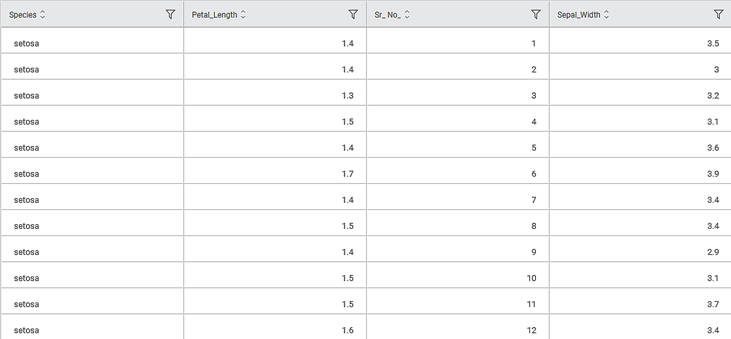
Consider the Iris dataset with columns of Sepal length, Sepal Width, Petal width and Species. A snippet of input data is shown in the figure below.

The selected values for properties and Advanced options for the Cumulative Distribution Function are given in the table below.

Property	Value
Input Column	Petal Width
Distribution type	Discrete
Distribution	Binomial
Number of Trials	10
Probability	0.5

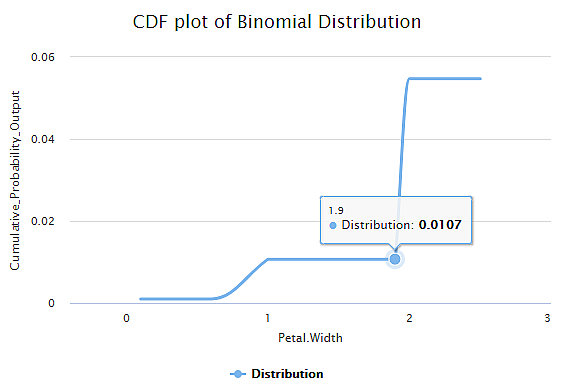
The Result page of the Cumulative Distribution Function is displayed in the figure below. The graph shows the variation in Cumulative Probability Output values for the given variable values (Petal Width) in the dataset.

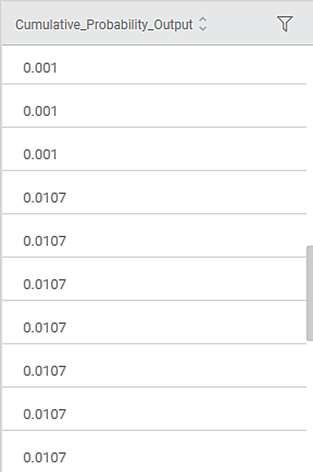
For example, the Petal Width value of 1.9 has a Cumulative Probability Output value of 0.0107.

The Data page of the Cumulative Distribution Function is displayed in the figure below. It shows a snippet of the Cumulative Probability Output values corresponding to Input Column values in tabular form. By default, the values of Petal Width are sorted and arranged in an ascending order.

Table of Contents